FINANCIAL MATURITY
A Guide to Increasing Financial Returns From Your Woodland
By Randall B. Heiligmann, Extension Specialist, Forestry
When a forest is harvested using either the individual tree or group selection method1, trees to be cut or retained are commonly selected based on a number of factors including species, quality, diameter, distance from other trees, health and vigor, non-timber value (e.g., wildlife, aesthetics, etc.), risk of loss or damage (during harvest or during the time interval before the next harvest), and maturity. Maturity may refer to either biological or financial maturity, depending on the landowner’s objectives.
Biological Maturity

Woodland owner evaluating financial maturity of white oak.
Biological maturity, as used here, refers to the age when a tree begins to decline in vigor and health and becomes increasingly susceptible to diseases and other harmful environmental factors that will ultimately result in death. This age of biological maturity, which might be referred to as a tree’s natural life expectancy, varies dramatically among species (even within genus) and with site quality. Red and silver maples, for example, often approach biological maturity well before they are 150 years old, while black and sugar maples are often still thriving well beyond 200 years of age. Other Ohio species, such as white oak, may live well beyond 300 years under favorable conditions. Trees growing on sites with less than favorable growing conditions generally have shorter life expectancies than those growing on more favorable sites. Landowners desiring large, stately trees, or who want their woodland to approximate an “old-growth” forest, define maturity primarily as biological. They want their trees to grow as large and live as long as possible and will probably only cut “mature” trees that constitute a safety hazard (extensive dead limbs, hollow, etc.).
Financial Maturity
In contrast, forest owners wishing to maximize the financial returns they receive from their woodland will be more concerned with financial maturity. Usually a tree is considered to be financially mature when its rate of value increase falls below a desired level. The rate of value increase of a tree can be determined by comparing the dollar value of its expected growth during a given time period (e.g., 10 years) with the dollar value of the tree prior to that growth. Think of the value of the tree as the principal in a bank account, and the value increase as the interest earned on that principal. This value increase can be expressed as an annual compound interest and compared with alternative investments or a desired rate of return. If the tree’s expected rate of value increase exceeds the desired rate, the tree is not financially mature and should be allowed to grow for the specified time period. If the tree’s expected rate of value increase is less than the desired rate, the tree is financially mature and, based on that criteria, should be cut.
Compound Interest Calculations.
The basic compound interest formula is
PV (1 + I)y = FV
Where PV = present value, I = compound interest rate, y = number of years interest compounded, and FV = future value of “PV” earning interest “I” for “y” years. If, for example, one wanted the value of $100 five years in the future if it was earning 5% compound interest annually, the answer would be
$100 (1.05)5 = FV = $127.63
The quantity (1.05)5 may be obtained by multiplying (1.05)(1.05)(1.05)(1.05)(1.05), or if your calculator has a yx key, by entering 1.05, then pressing the yx key, entering 5, and pressing the equals key.
Note that in our problem of rate of return earned by the yellow poplar tree, we need to solve for the interest rate (I). Using the equation presented previously
PV (1 + I)y = FV
$24(1 + I)10 = $51
(1 + I)10 = $51/$24
(1 + I)10 = 2.125
The equation now must be solved for the compound interest value “I” which satisfies the equality. This can be done several ways. One can try different interest rates until they zero in on the one that satisfies the equality. This will take some time. Again, if one has a calculator with a yx key and an INV key (INV stands for invert), the equation can quickly be solved for “I” by entering 2.125, pressing the INV key, then pressing the yx key, then entering 10, then pressing the = key. The answer that appears is 1.07829 which is the value of (1 + I). I is obtained by subtracting 1 from 1.07829 resulting in 0.07829 or 7.8%.
Calculating the Financial Maturity of an Individual Tree
Consider, for example, a yellow-poplar tree, 18-inch-dbh, containing two merchantable logs (32 feet), growing in diameter at a rate of five rings per radial inch, increasing in merchantable height at a rate of one-half log every 10 years, and having a current stumpage value of $150 per thousand board feet. According to a Doyle volume table2, the volume of an 18-inch-dbh tree containing two merchantable logs is 160 board feet. With a stumpage price of $150 per thousand board feet, this tree is worth $24. In the next 10 years, the tree is expected to grow four inches in diameter and increase one-half log in merchantable height, resulting in a 22-inch-dbh tree containing 2½ merchantable logs and 340 board feet. If the quality of the yellow-poplar remains essentially the same, the stumpage price of the tree will remain $150 per thousand (excluding inflation), and the stumpage value of this yellow-poplar in 10 years will be $51. The tree has increased in value $27, which represents an annual compound interest increase in value of 7.8% (see Figure 2 for calculations). If the woodland owner desired that the trees in the woodland increase in value at an annual rate of at least 5 percent (real rate of return excluding inflation), this yellow-poplar is certainly meeting that standard. It is not financially mature and should not be cut based on that criteria, but allowed to grow for at least the next 10 years.
Now suppose there is another yellow-poplar in the woodland that is also 18 inches in diameter and contains two merchantable logs and 160 board feet. However, it is growing in diameter at a rate of 10 rings per radial inch and will not put on any additional merchantable height due to branchiness. In 10 years, it is expected to be 20 inches in diameter and contain two merchantable logs and 220 board feet. Stumpage price (excluding inflation) will not increase because the tree will not improve enough in size or quality. The current stumpage value of the tree at a stumpage price of $150 per thousand is $24; the value after 10 years of growth is expected to be $33; and the annual compound interest increase in value of the tree over the 10-year period is 3.2%. If, as above, the woodland owner desires the trees to increase in value at a rate of at least 5%, this tree is not achieving that rate. Based on financial maturity criteria, this tree is financially mature and should not be allowed to grow for the next 10 years, but should be cut.
Notice that the quality of both of our yellow-poplar trees remained essentially the same during the time period between cuts. Often, however, the quality of the butt log improves enough that it is worth substantially more (higher stumpage price for butt log volume). What happens to our financial maturity analysis if this occurs? To answer this question, let’s look at another tree in our woodland, a 17-inch-dbh white oak that contains two merchantable logs, is growing at the rate of seven rings per radial inch, and will not increase further in merchantable height because of branching. Because this oak currently exhibits a number of branch scars and other minor defects on the butt log, the stumpage price is $400 per thousand board feet, and the tree, which contains 140 board feet, has a stumpage value of $56. In 10 years, the tree is expected to be 20 inches in diameter, still contain two merchantable logs, and contain 220 board feet. If, as with our yellow-poplar, the quality of the butt log does not change enough, the stumpage price will remain $400 per thousand board feet (excluding inflation), the stumpage value of the tree will be $88, and it will have increased in value at a rate of 4.6% over the 10-year period. If the woodland owner desired that the trees in the woodland increase in value at an annual rate of at least 5 percent, this oak is not meeting that standard. Based on financial maturity criteria, it is financially mature and should not be left to grow for the 10-year period.
But now suppose that the butt log is expected to increase in quality enough in the next 10 years to justify a stumpage price of $650 per thousand board feet. Increases in quality of butt logs are quite common in trees of this size in well-managed stands. As the tree increases in diameter, clear wood covers the branch stubs, branch scars, and other defects, producing a log relatively free of grade-reducing defects. At the end of the 10-year period, the oak is again expected to contain 220 board feet, but with 130 board feet in the butt log (board foot content of a 200-inch-dbh tree containing one merchantable log) worth $650 per thousand board feet and 90 board feet in the second log (220 board feet in the total tree minus 130 board feet in the first log) worth $400 per thousand. The total expected stumpage value of the oak in 10 years is $120.50, and it is increasing in value at a rate of 8% per year. Based on our financial maturity criteria of 5%, this oak should remain in the stand for the 10-year period.
Obviously, calculating the financial maturity of a tree is a complex process that involves estimating many variables, including current stumpage value, diameter, and merchantable height growth rate, possible changes in tree quality, and future stumpage value. Often calculations of financial maturity for a particular tree must be done separately for the butt log and the remainder of the merchantable height because of differences in value. The process can be further complicated by recognizing that the decision not to cut a tree delays for some period of time the establishment of future trees. This delay has a cost that can be calculated and that can be an important factor in some situations. That calculation, however, is beyond the scope of this article.
A Tabular Guideline to Financial Maturity
Because of the complexity of performing financial maturity calculations, these calculations are not often undertaken by forest landowners. It is important, nevertheless, for anyone involved in managing uneven-aged stands to have an understanding of the concept of financial maturity and some grasp of the rate of return being earned by trees of different species and different sizes. Some landowners and many foresters will calculate financial maturities. Others can gain some valuable insight and general guidelines from a study by Trimble, Mendel and Kennell3 in which they present financial maturity guidelines based on their research in uneven-aged forests (see Table 1).
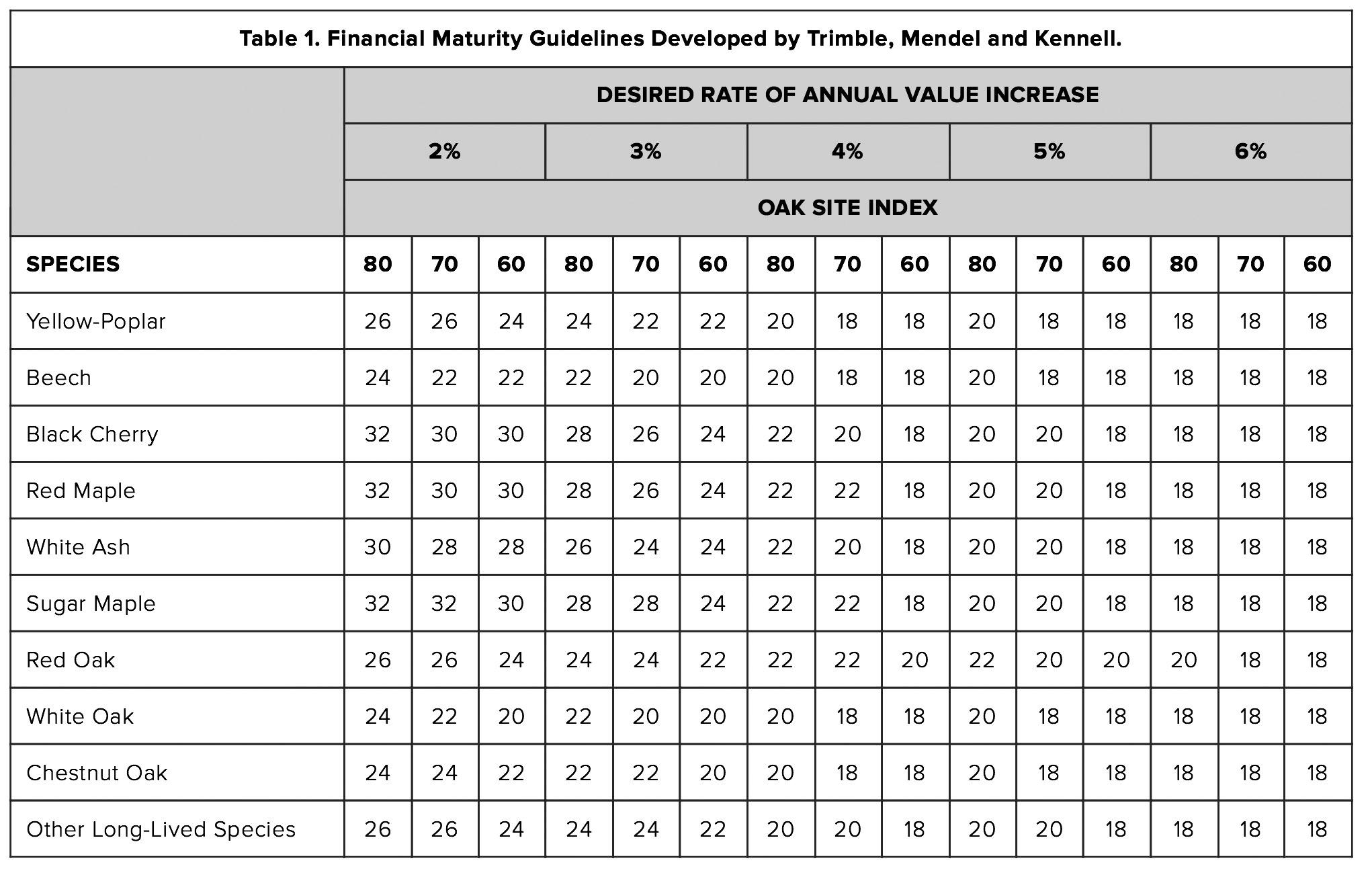 The numbers in the table are tree diameters (2-inch-dbh classes). The table indicates the 2-inch diameter class of each species that will no longer earn the rate-of-return listed at the top of the table. Using the table as a guide to financial maturity, a landowner desiring an annual increase in value of 4 percent would cut an 18-inch black cherry growing on an oak site index 60 unless the butt log was going to increase in grade, but would retain the same tree on a site index 70 or 80. When interpreting this table, it is important to be aware that the table assumes no increase in the grade of the butt log in the tree. According to the authors’ research, if the butt log will increase in grade within the period of evaluation (10 years in our examples), it is usually not financially mature and should not be cut. In their article, Trimble, Mendel and Kennell make several observations about financial maturity that are worth noting and thinking about.
The numbers in the table are tree diameters (2-inch-dbh classes). The table indicates the 2-inch diameter class of each species that will no longer earn the rate-of-return listed at the top of the table. Using the table as a guide to financial maturity, a landowner desiring an annual increase in value of 4 percent would cut an 18-inch black cherry growing on an oak site index 60 unless the butt log was going to increase in grade, but would retain the same tree on a site index 70 or 80. When interpreting this table, it is important to be aware that the table assumes no increase in the grade of the butt log in the tree. According to the authors’ research, if the butt log will increase in grade within the period of evaluation (10 years in our examples), it is usually not financially mature and should not be cut. In their article, Trimble, Mendel and Kennell make several observations about financial maturity that are worth noting and thinking about.
- The rate of tree earning power in percent is less for trees of large diameter, even though actual dollar earning will often be greater for the larger trees. Remember that the larger diameter trees contain higher volumes and represent a greater principal on which the value increase is earned.
- The rate of earning power in percent is lower for trees of present high quality, even though these trees may have higher dollar earnings. The higher-quality trees represent a greater principal on which the value increase is earned.
- A tree’s earning power is greatly increased by improvement in tree quality (grade).
- A tree’s earning power increases markedly with increasing diameter growth rate.
- Trees with greater merchantable height usually have slightly higher earning power.
- Trees that increase in merchantable height have higher earning power, providing the upper logs have value.
Two additional useful observations can be made from the table.
- At low rates of return, there are dramatic differences among species in the diameter of a financially mature tree. These differences disappear as rates of return increase.
- Site quality affects the financial maturity size (diameter) of trees. On better sites, where growth rates are higher, larger trees are left for any desired rate of return. In Table 1, for example, if 4 percent is the desired annual growth rate, an 18-inch-dbh white oak on an oak site index 60 or 70 would be judged financially mature and be cut unless the butt log was going to increase in grade before the next harvest. On the other hand, an 18-inch-dbh white oak growing on an oak site index 80 would be evaluated as not financially mature and would be retained until the next harvest, based on financial maturity criteria.
Closing Thoughts
Financial maturity analysis is an analytical method of evaluating the financial value of a tree in terms of its potential value increase. It can be used by woodland owners as a financial criteria to identify which trees to cut in a harvest and which to retain, or to identify income foregone when trees are grown beyond their financial maturity in order to achieve nonfinancial ownership objectives (economists call this an opportunity cost). When used as a criteria to determine which trees to cut and which to retain in a harvest, it is extremely important to emphasize that financial maturity is only one factor to be evaluated. As enumerated at the beginning of this article, other factors must be considered when making such an evaluation, including species, quality, diameter, distance from other trees, health and vigor, non-timber value (e.g., wildlife, aesthetics, etc.), and risk of loss or damage (during harvest or during the time interval before the next harvest). If financial maturity alone is used as a marking guide, the marking and subsequent harvest becomes little more than a refined diameter-limit cutting.

